Tutorial Week 3

Integration Methods Review

Q1: Integrate \(\int sin^4(x) dx\).

Show Solution

Trigonometric Substitution (Trig Sub)

The method of Trig sub takes advantage of your trig identities to simplify integrals and in a way, it’s just fancy and strategic U-sub. Refer to the following table for which substitution to use:

Expression	\(\sqrt{a^2-x^2}\)	\(\sqrt{a^2+x^2}\)	\(\sqrt{x^2-a^2}\)
Substitution	\(x=a\sin \theta\)	\(x=a\tan \theta\)	\(x=a\sec \theta\)
Domain	\(\theta \in [-\frac{\pi}{2}, \frac{\pi}{2}]\)	\(\theta \in (-\frac{\pi}{2}, \frac{\pi}{2})\)	\(\theta \in [0, -\frac{\pi}{2}) \cup (\frac{\pi}{2}, \pi]\)

Q2: Integrate \(\int \frac{1}{\sqrt{x^2 + 10x + 27}} dx\).

Show Solution

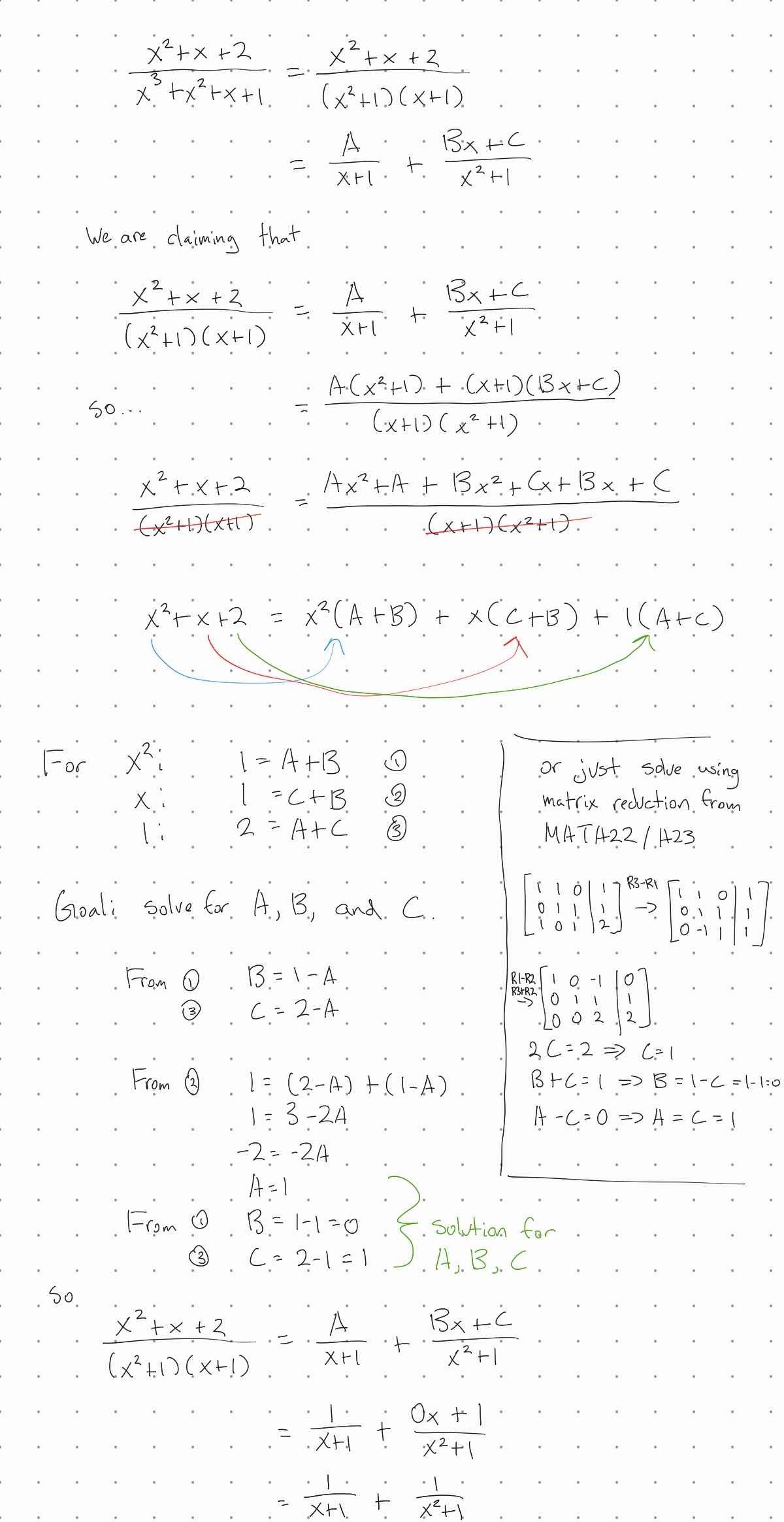
Partial Fraction Decomposition (PFD)

Partial fraction decomposition is a general algebraic method of rewriting a fraction as a sum of simpler fractions.

There are 2 steps to finding PFDs:

1. Fully factor the denominator and write the partial factors

2. Solve for the variables in the partial factors

For the first step, the partial factors for each demoniator factor follows the pattern of:

Form of Denominator Factor	Partial Factor
\(\frac{1}{ax + b}\)	\(\frac{A}{ax+b}\)
\(\frac{1}{(ax + b)^2}\)	\(\frac{A}{ax+b} + \frac{B}{(ax+b)^2}\)
\(\frac{1}{ax^2 + bx + c}\)	\(\frac{Ax+B}{ax^2+bx+c}\)
\(\frac{1}{(ax^2 + bx + c)^2}\)	\(\frac{Ax+B}{ax^2+bx+c} + \frac{Cx+D}{(ax^2+bx+c)^2}\)

The complete partial factors for a fraction will be a sum of each of the individual partial factors for each denominator factor.

Q3: Find the PFD of \(\frac{x^{2}+x+2}{x^{3}+x^{2}+x+1}\).

Show Solution