Tutorial Week 6
Volumes of Revolution
The volume of a solid generated by revolving a function \(f(x)\) around the x axis on the interval \([a, b]\) is given by \(\int_a^b \pi f(x)^2 dx\).
Q1: Find the volume created by rotating the region bounded between \(y =3-x^{2}\) and \(y =2x^{2}\) on the interval \(x\in[0, 1]\) about the x-axis.
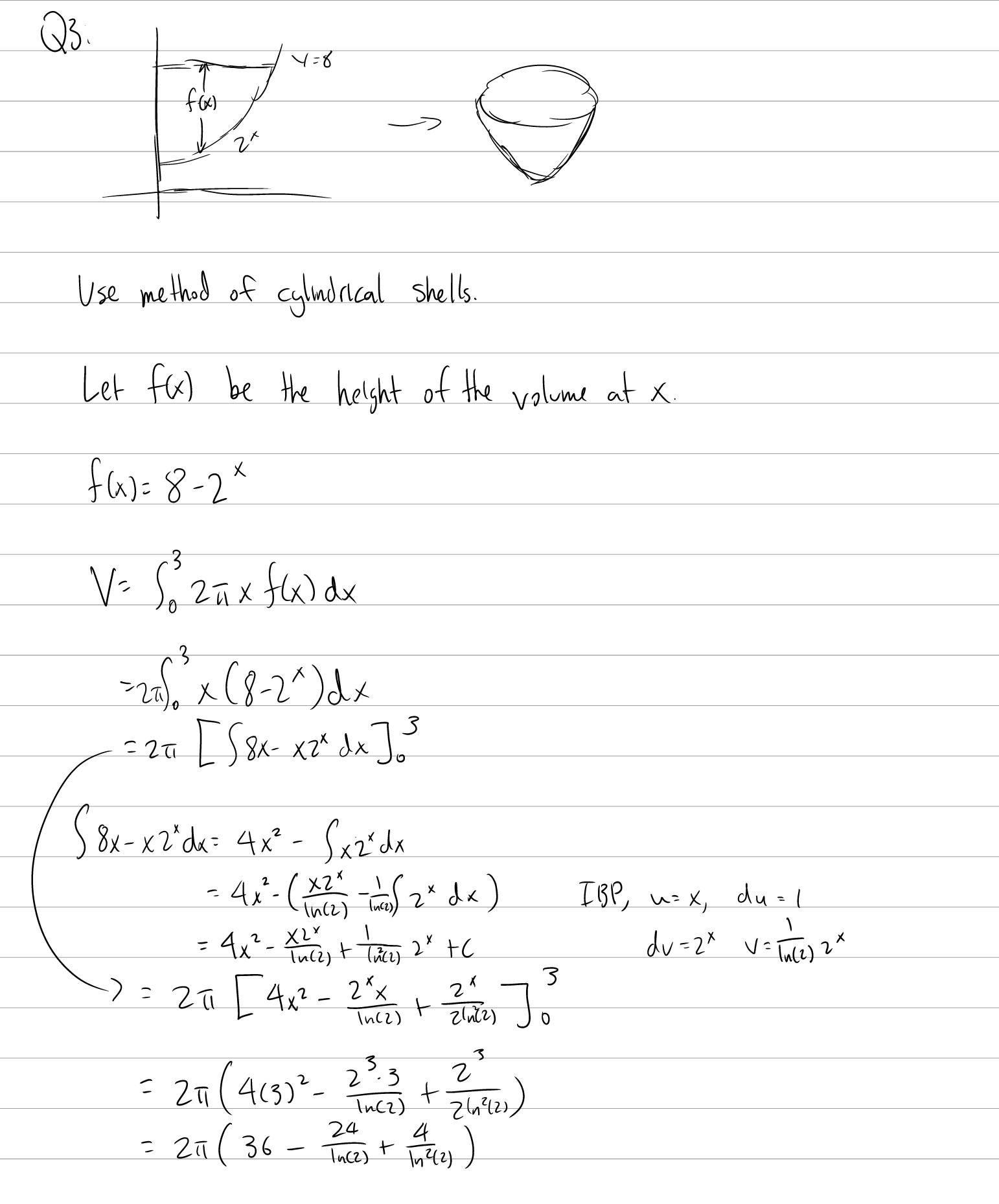
Volumes by Cylindrical Shells
The volume of a solid generated by revolving a function \(f(x)\) around the y axis on the interval \([a, b]\) is given by \(\int_a^b 2x\pi f(x) dx\).
Q2: Find the volume created by rotating the region bounded below by \(y = 2^x\) and bounded above by \(y = 8\) on \(x \in [0, 3]\) about the y-axis.
